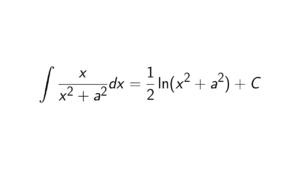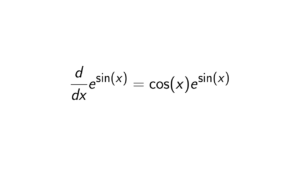Let R be a ring with identity 1 \neq 0. The commutative ring R is a field iff its only ideals are 0 and R
Proof. We know that a commutative ring R is a field if and only if every nonzero element of R is a unit. So now can translate the question as the following:
Every nonzero element of R is a unit iff its only ideals are 0 and R
“\Rightarrow“: Assume we have a proper ideal I of R which is not equal to the zero ideal. Then that ideal I contains a unit element of R, say u \in I. Since I is an ideal of R, we have that Ru \subseteq I. This implies that 1 \in I since there exists a v \in R such that vu = 1. Therefore, I = R and can’t be a proper ideal of R.
“\Leftarrow“: Assume that the only ideals of R are 0 and R. Take an arbitrary nonzero element x\in R. We want to prove that x is a unit. Since the only ideals are 0 and R, we have that the ideal (x) is equal to R. So we get that 1 \in (x). Therefore, there exist an y \in R such that xy = 1, and so x is a unit of R. Since x is taken arbitrarily, we have that every nonzero element of R is a unit.