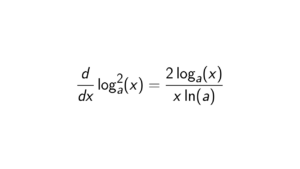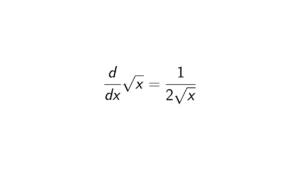Prove that the center of a ring is a subring that contains the identity.
Proof. Recall that the center of a ring
R is defined as:
\begin{align*}
Z = \{z \in R \ | \ zr = rz \ \text{for all} \ r \in R\}.
\end{align*}
Firstly, note that
Z is not empty since it contains the identity
1 (1z = z1).
Secondly, we need to show that
Z is closed under subtraction. Let
z_1,z_2 \in Z, then
z_1r = rz_1 and
z_2r = rz_2 for all
r \in R. We get the following:
\begin{align*}
(z_1 - z_2)r &= z_1r - z_2r \\
&= rz_1 - rz_2 \\
&= r(z_1 - z_2),
\end{align*}
which implies that
z_1 - z_2 \in Z. So,
Z is closed under subtraction.
What is left is that
Z is closed under multiplication. Take again
z_1,z_2 \in Z. Then we need to show that
z_1z_2 \in Z. Notice that
\begin{align*}
z_1r = rz_1 \quad \text{and} \quad z_2r = rz_2.
\end{align*}
We need to show that
z_1z_2r = rz_1z_2 holds:
\begin{align*}
z_1z_2r = z_1rz_2 \iff z_1z_2r = rz_1z_2
\end{align*}
since
z_1r = rz_1 and
z_2r = rz_2.
Therefore, the center of a ring is a subring that contains the identity.H