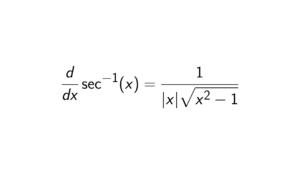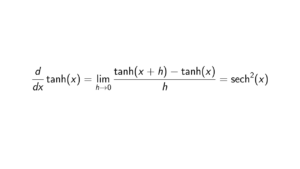How to prove that a subring R of the PID R[x] is an integral domain

The reader should know that only proving that the subring has no zero divisors is not enough. A subring should also contain the identity element.

Prove that a subring R of the PID R[x] is an integral domain

Proof: we take the principal ideal domain R[x] and let R be its subring. Let’s assume that we have the non-zero elements a,b \in R such that ab = 0, i.e., a and b are zero divisors. Since R \subset R[x], we have that ab = 0, but that is a contradiction since R[x] has no zero divisors since R[x] is an integral domain.

Now we need to figure out if R contains the identity element. But, R[x] contains the identity element since it is a principal ideal domain, and therefore, R too (so 1_R \neq 0_R).