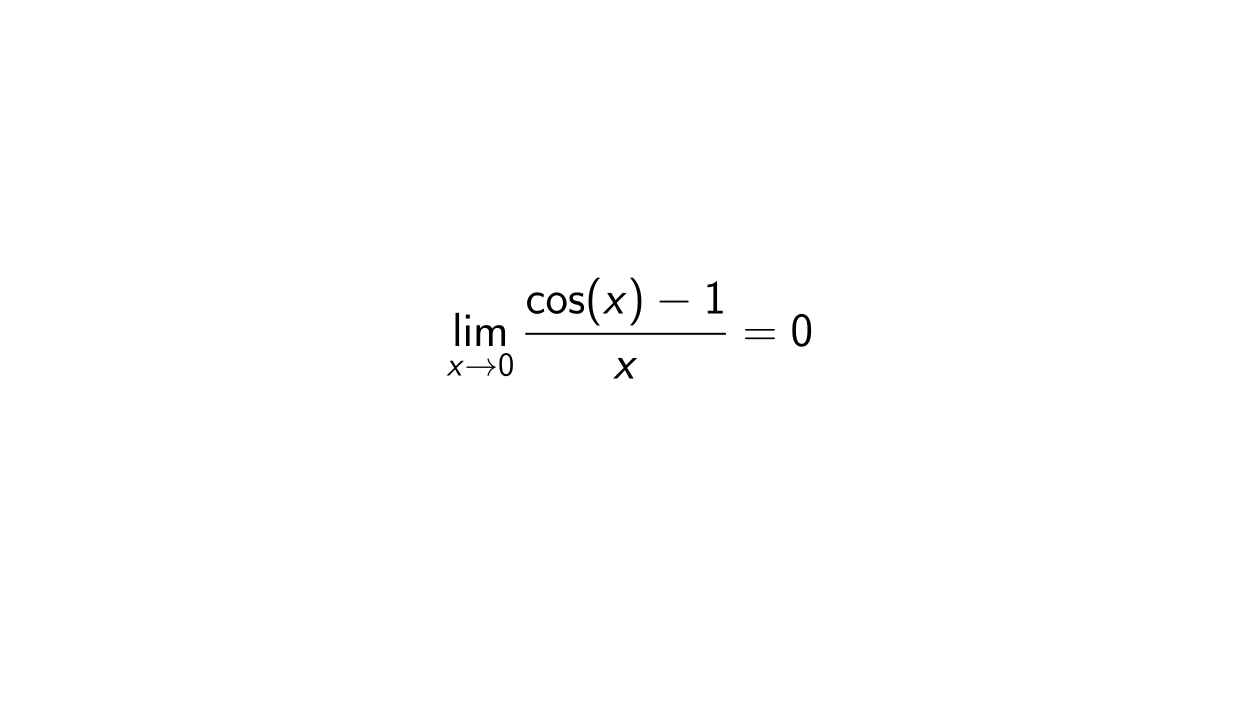Determine the limit (cos(x)-1)/x as x approaches 0

We will prove that the limit of

(\cos(x) - 1)/x

x

approaches 0 is equal to 0. We will prove that in two different ways.

Proof. We would like to prove the next limit:

\begin{equation*} \lim_{x \rightarrow 0}\frac{\cos(x) - 1}{x} = 0 \end{equation*}

We do have the next identity:

\begin{equation*} \cos(x) = 1 - 2\sin^2(x/2) \end{equation*}

Therefore, we get

\begin{align*} \lim_{h \rightarrow 0}\frac{\cos(x) - 1}{x} &= \lim_{x \rightarrow 0}\frac{- 2\sin^2(x/2)}{x} \\ &= \lim_{x \rightarrow 0}\frac{- 2\sin^2(x/2)}{x} \\ &= -\lim_{x \rightarrow 0}\frac{\sin(x/2)}{x/2} \cdot \lim_{x \rightarrow 0} sin(x/2) \\ &= (-1) \cdot 0 = 0. \end{align*}

where

\lim_{x \rightarrow 0}\frac{\sin(x/2)}{x/2} = 1

which is proved in this article. Another way to prove this is to use the Maclaurin series. We know that

\begin{align*} \cos(x) &= \sum_{n = 0}^{\infty} \frac{(-1)^n}{(2n)!}x^{2n} \\ &= 1 - \frac{x^2}{2!} + \frac{x^4}{4!} - \cdots \end{align*}

Now we have that

\cos(x) - 1 = - \frac{x^2}{2!} + \frac{x^4}{4!} - \cdots

. Therefore, we get

\begin{align*} \lim_{x \rightarrow 0}\frac{\cos(x) - 1}{x} &= \lim_{x \rightarrow 0}\frac{- \frac{x^2}{2!} + \frac{x^4}{4!} - \cdots}{x} \\ &= \lim_{x \rightarrow 0} - \frac{x}{2!} + \frac{x^3}{4!} - \cdots \\ &= 0 \end{align*}

which completes the proof.