What Are The Conjugacy Classes of S3?

What are the conjugate classes of $S_3$

Proof. We know that

\begin{equation*} S_3 = \{e,(12),(13),(23),(123),(132)\}. \end{equation*}

To determine the conjugates of the element

\tau \in S_3

, we need to show that

\sigma \tau \sigma^{-1}

for all

\sigma \in S_3

. So we will check one by one the conjugates of the elements of the symmetric group

S_3

: $\tau = e$

\begin{align*} eee &= e \\ (12)e(21) &= e \\ (13)e(31) &= e \\ (23)e(32) &= e \\ (123)e(321) &= e \\ (132)e(231) &= e \\ \end{align*}

$\tau = (12)$

\begin{align*} e(12)e &= (12) \\ (12)(12)(21) &= (12) \\ (13)(12)(31) &= (23) \\ (23)(12)(32) &= (13) \\ (123)(12)(321) &= (23) \\ (132)(12)(231) &= (13) \\ \end{align*}

$\tau = (13)$

\begin{align*} e(13)e &= (13) \\ (12)(13)(21) &= (23) \\ (13)(13)(31) &= (13) \\ (23)(13)(32) &= (12) \\ (123)(13)(321) &= (12) \\ (132)(13)(231) &= (23) \\ \end{align*}

$\tau = (23)$

\begin{align*} e(23)e &= (23) \\ (12)(23)(21) &= (13) \\ (13)(23)(31) &= (12) \\ (23)(23)(32) &= (23) \\ (123)(23)(321) &= (13) \\ (132)(23)(231) &= (12) \\ \end{align*}

$\tau = (123)$

\begin{align*} e(123)e &= (123) \\ (12)(123)(21) &= (132) \\ (13)(123)(31) &= (132) \\ (23)(123)(32) &= (132) \\ (123)(123)(321) &= (123) \\ (132)(123)(231) &= (123) \\ \end{align*}

$\tau = (132)$

\begin{align*} e(132)e &= (132) \\ (12)(132)(21) &= (123) \\ (13)(132)(31) &= (123) \\ (23)(132)(32) &= (123) \\ (123)(132)(321) &= (132) \\ (132)(132)(231) &= (132) \\ \end{align*}

We see that we have the next conjugacy classes of

S_3

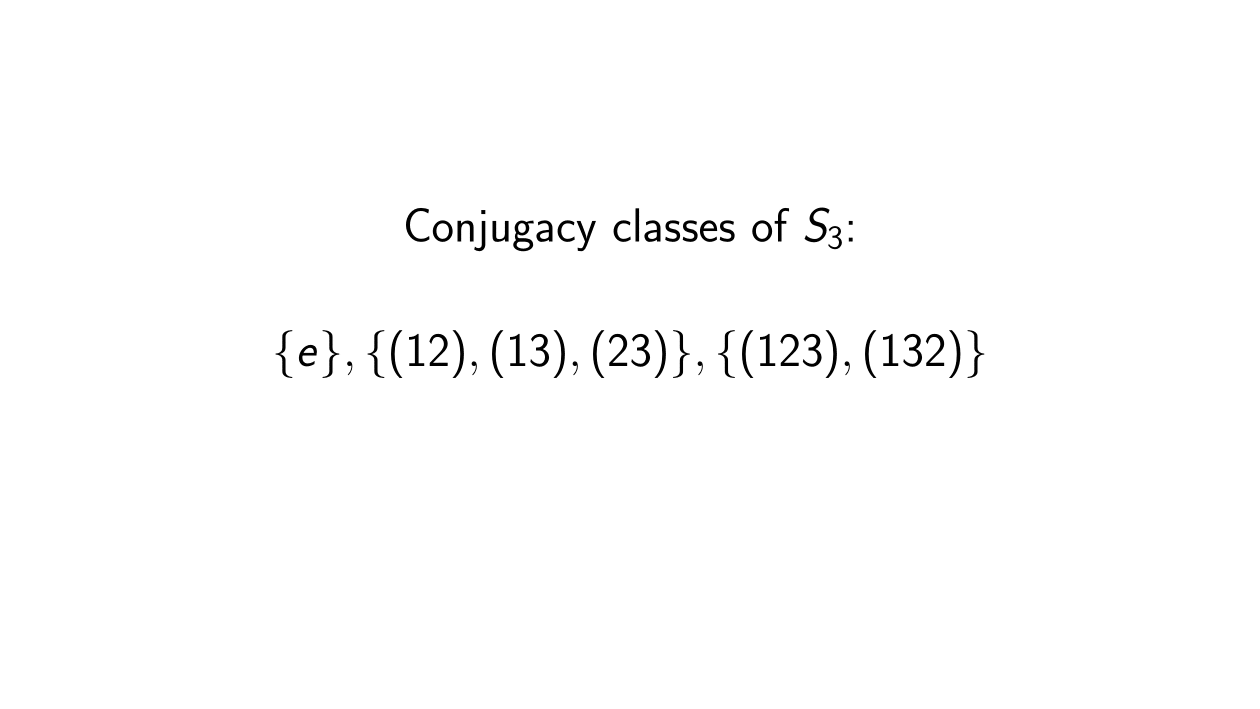
\begin{equation*} \{e\}, \{(12),(13),(23)\}, \{(123),(132)\} \end{equation*}

What are the Conjugacy Classes of S3