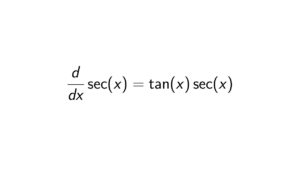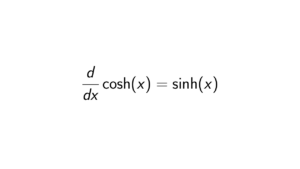Define the following mapping:
\begin{align*}
f: \mathbb{Z} &\longrightarrow \langle x \rangle \\
k &\longmapsto x^k,
\end{align*}Proof. To prove that the map f is isomorphic, we need to show that f:
- it is well defined,
- is injective,
- is surjective,
- and is a homomorphism.
Injectivity. All elements are distinct from what we have seen before, so if x^a \neq x^b, then a \neq b.
Surjectivity. The element x^k of \langle x \rangle is the image of k under f, so f is surjective.
Homomorphism. To prove that f is a homomorphism, we have done that here. Now we have shown that every infinite cyclic group is isomorphic to the additive group of Z.