Solution. We want to determine the integral of e^{-x}, i.e.:
\begin{align*}
\int \frac{1}{e^x} dx = \int e^{-x}dx.
\end{align*}\begin{align*}
\int e^{-x} dx &= - \int e^u du \\
&= -e^u + C \\
&= -e^{-x} + C.
\end{align*}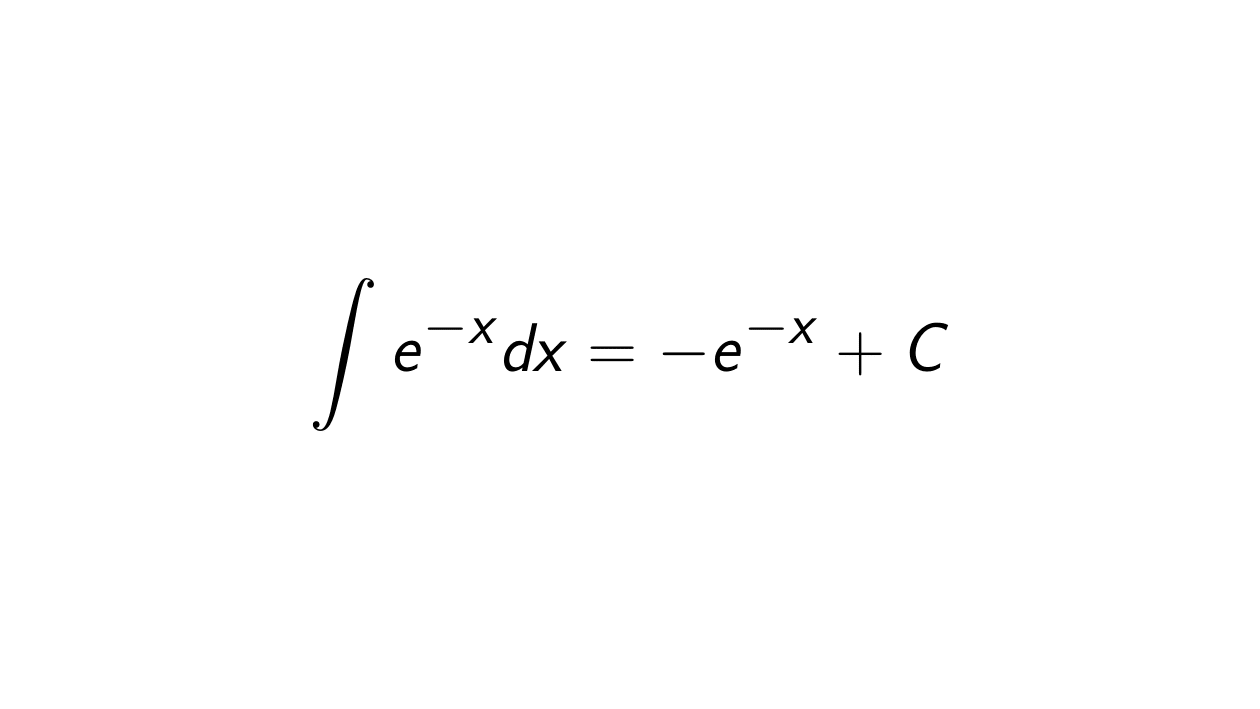
\begin{align*}
\int \frac{1}{e^x} dx = \int e^{-x}dx.
\end{align*}\begin{align*}
\int e^{-x} dx &= - \int e^u du \\
&= -e^u + C \\
&= -e^{-x} + C.
\end{align*}