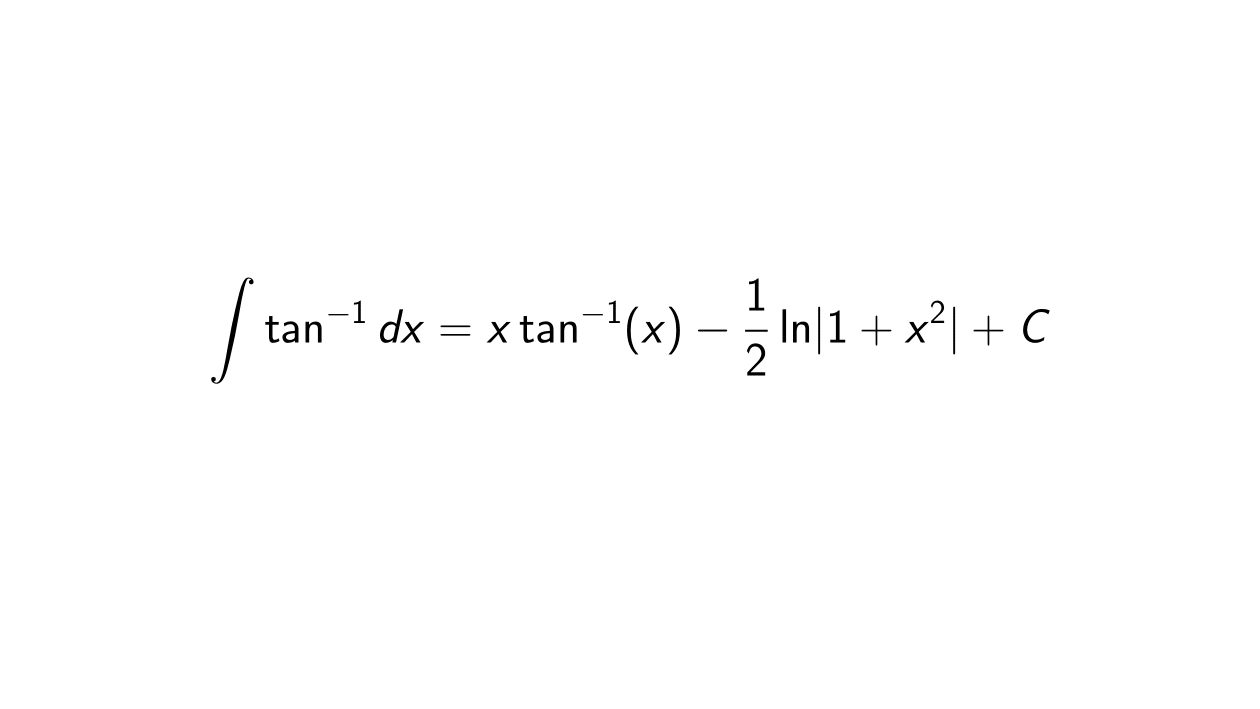What is the integral of arctan(x)?

integral of arctan(x)

The integral of

\tan^{-1}(x)

x\tan^{-1}(x) - \frac{1}{2}\ln \lvert 1 + x^2 \rvert + C

.

Solution. We want to find the integral of

\tan^{-1}(x)

, i.e.:

\begin{align*} \int \tan^{-1}(x) dx. \end{align*}

Now we will use the method integrating by parts, i.e.:

\begin{align*} \int UdV = UV - \int VdU, \end{align*}

where we get the following functions:

\begin{align*} U = \tan^{-1}(x), \quad &dV = dx\\ dU = \frac{dx}{1 + x^2}, \quad &V = x. \end{align*}

To see why

dU

is true, see the solution here. So we get:

\begin{align*} \int \tan^{-1}(x) dx = x\tan^{-1}(x) - \int \frac{x}{1 + x^2} dx. \end{align*}

The last method we will use is the substitution method. Let

u = 1 + x^2

, then

du = 2x dx

. Combining everything, we get:

\begin{align*} \int \tan^{-1}(x) dx &= x\tan^{-1}(x) - \int \frac{x}{1 + x^2} dx \\ &= x\tan^{-1}(x) - \frac{1}{2} \int \frac{1}{u} du \\ &= x\tan^{-1}(x) - \frac{1}{2} \ln\lvert u \rvert + C \\ &= x\tan^{-1}(x) - \frac{1}{2} \ln\lvert 1 + x^2 \rvert + C. \end{align*}

Therefore, the integral of

\tan^{-1}(x)

x\tan^{-1}(x) - \frac{1}{2}\ln \lvert 1 + x^2 \rvert + C