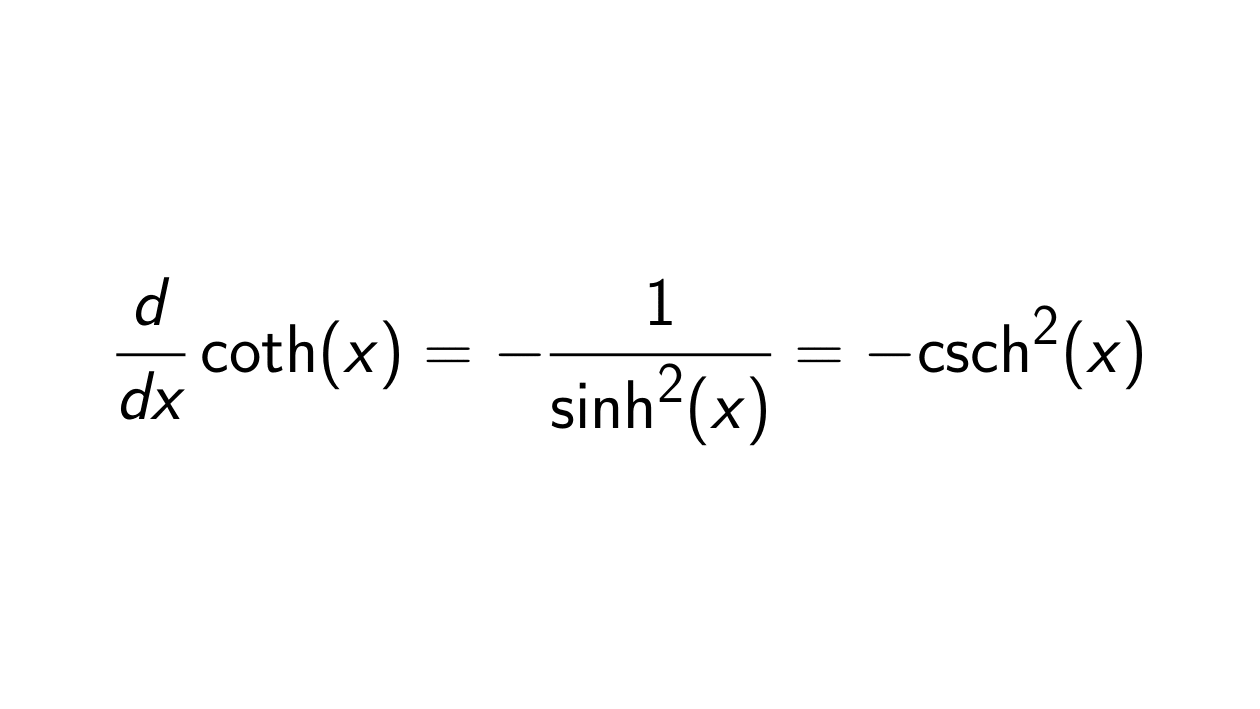What is the Derivative of Hyperbolic Cotangent?

Derivative of Hyperbolic Cotangent

The derivative of

\coth(x)

-\text{csch}^2(x)

.

Solution. We know that

f(x) = \coth(x) = \frac{\cosh(x)}{\sinh(x)} = \frac{e^x + e^{-x}}{e^x - e^{-x}}

. So there are two different ways to prove this. The first way is to do it via

f(x) = \coth(x) = \frac{\cosh(x)}{\sinh(x)} = \frac{g(x)}{h(x)}

. We will apply the quotient rule here:

\begin{align*} f'(x) = \frac{d}{dx} \coth(x) = \frac{\cosh(x)}{\sinh(x)} = \frac{g(x)}{h(x)} = \frac{g'(x)h(x) - g(x)h'(x)}{h(x)^2}. \end{align*}

Since

g'(x) = \frac{d}{dx} \cosh(x) = \sinh(x)

and

h'(x) = \frac{d}{dx} \sinh(x) = \cosh(x)

, we have that

\begin{align*} f'(x) &= \frac{d}{dx} \coth(x) \\ &= \frac{\cosh(x)}{\sinh(x)} \\ &= \frac{\sinh(x)\sinh(x) - \cosh(x)\cosh(x)}{\sinh^2(x)} \\ &= \frac{\sinh^2(x) - \cosh^2(x)}{\sinh^2(x)} \\ &= \frac{-1}{\sinh^2(x)} \\ &= -\text{csch}^2(x). \end{align*}

So the derivative of

\coth(x)

-\text{csch}^2(x)

. The second way is to do it by

f(x) = \coth(x) = \frac{\cosh(x)}{\sinh(x)} = \frac{e^x + e^{-x}}{e^x - e^{-x}}

, which we leave to the reader.