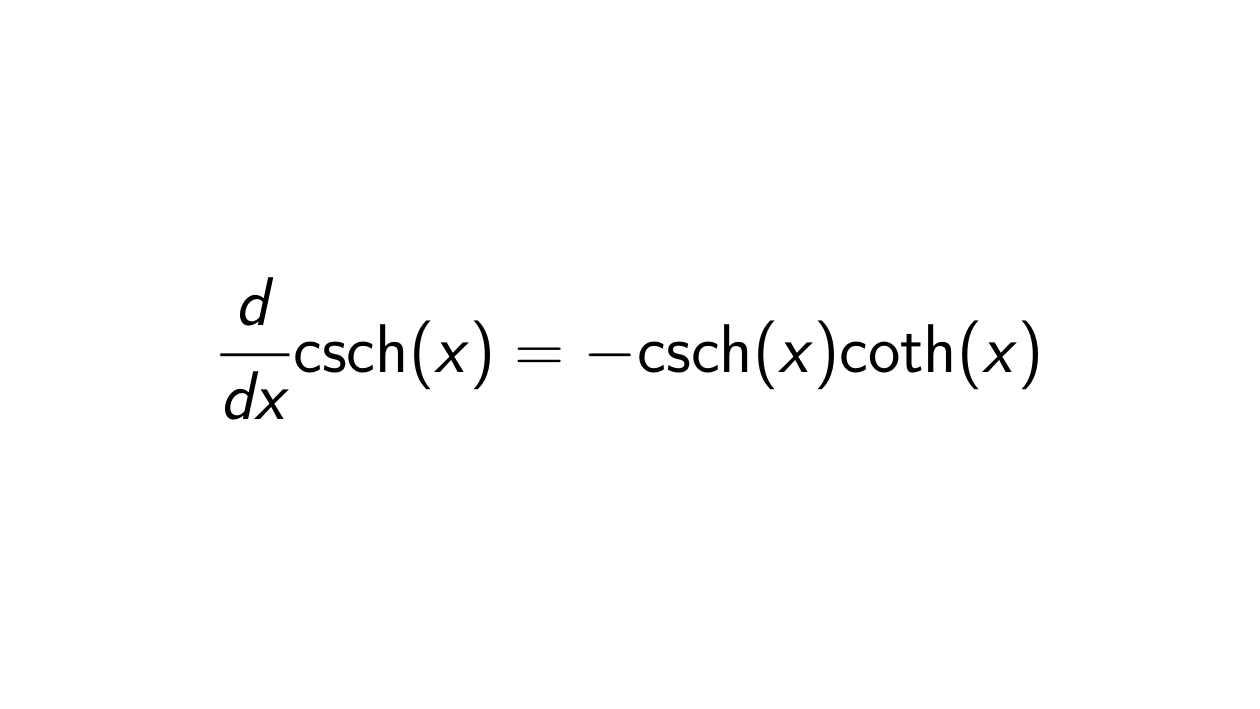Solution. Let F(x) = \text{csch}(x) = \frac{2}{e^x - e^{-x}} where f(u) = \frac{2}{u} and g(x) = e^x - e^{-x} such that F(x) = f(g(x)). Then we will use the chain rule to find the derivative of \text{csch}(x):
\begin{align*}
F'(x) = f'(g(x))g'(x).
\end{align*}\begin{align*}
f'(g(x)) = \frac{-2}{g(x)^2} = \frac{-2}{(e^x + e^{-x})^2}.
\end{align*}\begin{align*}
F'(x) &= f'(g(x))g'(x) \\
&= \frac{-2}{(e^x - e^{-x})^2} \cdot (e^x + e^{-x}) \\
&= \frac{-2(e^x + e^{-x})}{(e^x - e^{-x})^2} \\
&= \frac{-2}{e^x - e^{-x}} \cdot \text{coth}(x) \\
&= - \text{csch}(x)\text{coth}(x).
\end{align*}