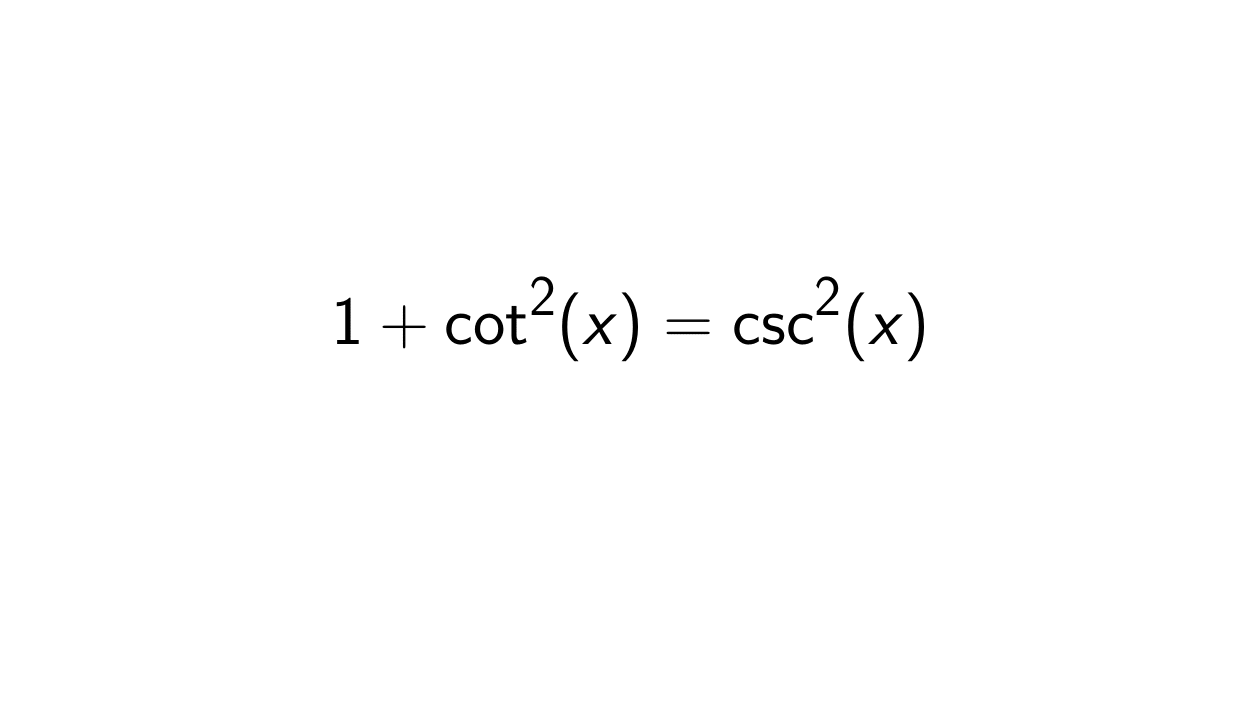Prove that 1 + cot^2(x) = csc^2(x)

1 + cot^2(x) = csc^2(x)

The function

1 + \cot^2(x)

is equal to

\csc^2(x)

.

Proof. We have seen here that

\sin^2(x) + \cos^2(x) = 1

. We can change that into the following:

\begin{align*} \sin^2(x) + \cos^2(x) = 1 &\iff \frac{1}{\sin^2(x)}(\sin^2(x) + \cos^2(x)) = \frac{1}{\sin^2(x)}\cdot 1 \\ &\iff \frac{\sin^2(x)}{\sin^2(x)} + \frac{\cos^2(x)}{\sin^2(x)} = \frac{1}{\sin^2(x)} \\ &\iff 1 + \frac{\cos^2(x)}{\sin^2(x)} = \frac{1}{\sin^2(x)}. \end{align*}

As we see, we multiplied

\sin^2(x) + \cos^2(x) = 1

both sides with

\frac{1}{\sin^2(x)}

. Further, we know that

\frac{\cos^2(x)}{\sin^2(x)} = \cot^2(x)

and

\frac{1}{\sin^2(x)} = \csc^2(x)

. So we have:

\begin{align*} 1 + \cot^2(x) = \csc^2(x). \end{align*}

Therefore,

1 + \cot^2(x)

is equal to

\csc^2(x)