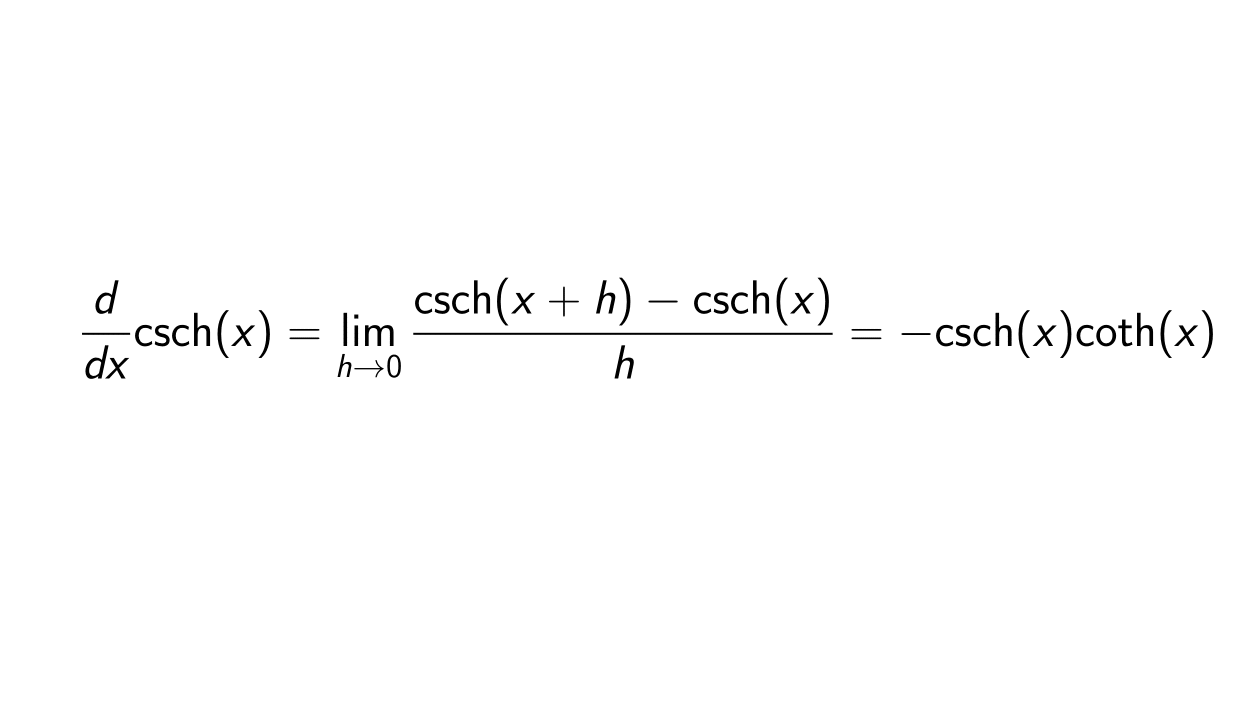Proof. Let f(x) = \text{csch}(x) = \frac{2}{e^x - e^{-x}}. Then we use the first principle of derivatives to determine \text{sech}(x):
\begin{align*}
f'(x) &= \lim_{h \rightarrow 0} \frac{f(x + h) - f(x)}{h} \\
&= \lim_{h \rightarrow 0} \frac{\text{csch}(x + h) - \text{csch}(x)}{h} \\
&= \lim_{h \rightarrow 0} \frac{\frac{2}{e^{x + h} - e^{-x - h}} - \frac{2}{e^x - e^{-x}}}{h} \\
&= \lim_{h \rightarrow 0} \frac{\frac{2e^{x} - 2e^{-x} - 2e^{x + h} + 2e^{-x - h}}{(e^{x + h} - e^{-x - h})(e^{x} - e^{-x})}}{h} \\
&= 2 \cdot \lim_{h \rightarrow 0} \frac{\frac{e^{x} - e^{-x} - e^{x + h} + e^{-x - h}}{(e^{x + h} - e^{-x - h})(e^{x} - e^{-x})}}{h} \\
&= \frac{2}{e^{x} - e^{-x}} \cdot \lim_{h \rightarrow 0} \frac{\frac{e^{x} - e^{-x} - e^{x + h} + e^{-x - h}}{e^{x + h} - e^{-x - h}}}{h} \\
&= \text{csch}(x) \cdot \lim_{h \rightarrow 0} \frac{\frac{e^{x} - e^{-x} - e^{x + h} + e^{-x - h}}{e^{x + h} - e^{-x - h}}}{h} \\
&= \text{csch}(x) \cdot \lim_{h \rightarrow 0} \frac{e^{x} - e^{-x} - e^{x + h} + e^{-x - h}}{h(e^{x + h} - e^{-x - h})} \\
&= \text{csch}(x) \cdot \lim_{h \rightarrow 0} \frac{1}{e^{x + h} - e^{-x - h}} \cdot \lim_{h \rightarrow 0} \frac{e^{x} - e^{-x} - e^{x + h} + e^{-x - h}}{h} \\
&= \text{csch}(x) \cdot \frac{1}{e^{x} - e^{-x}} \cdot \lim_{h \rightarrow 0} \frac{e^{x} - e^{-x} - e^{x + h} + e^{-x - h}}{h} \\
&= \text{csch}(x) \cdot \frac{1}{e^{x} - e^{-x}} \cdot \lim_{h \rightarrow 0} \frac{e^{x}(1 - e^{h}) - e^{-x}(1 - e^{-h})}{h} \\
&= -\text{csch}(x) \cdot \frac{1}{e^{x} - e^{-x}} \cdot \lim_{h \rightarrow 0} \frac{e^{x}(e^{h} - 1) - e^{-x}(e^{-h} - 1)}{h}. \\
\end{align*}\begin{align*}
\lim_{h \rightarrow 0} \frac{e^{x}(e^{h} - 1) - e^{-x}(e^{-h} - 1)}{h} &= \lim_{h \rightarrow 0} \frac{e^{x}(e^{h} - 1)}{h} - \lim_{h \rightarrow 0} \frac{e^{-x}(e^{-h} - 1)}{h} \\
&= e^{x} \cdot \lim_{h \rightarrow 0} \frac{(e^{h} - 1)}{h} - e^{-x} \cdot \lim_{h \rightarrow 0} \frac{(e^{-h} - 1)}{h} \\
\end{align*}\begin{align*}
\lim_{h \rightarrow 0} \frac{e^{x}(e^{h} - 1) - e^{-x}(e^{-h} - 1)}{h} = e^{x} + e^{-x}.
\end{align*}\begin{align*}
f'(x) &= -\text{csch}(x) \cdot \frac{1}{e^{x} - e^{-x}} \cdot (e^{x} + e^{-x}) \\
&= -\text{csch}(x) \cdot \frac{e^{x} + e^{-x}}{e^{x} - e^{-x}} \\
&= -\text{csch}(x) \text{coth}(x).
\end{align*}