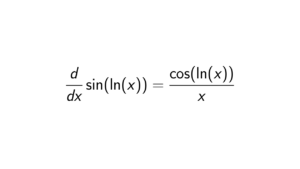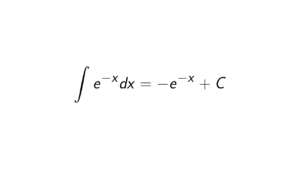How to prove reciprocal rule derivative using first principle of derivatives?
We will prove the reciprocal rule by the first principle method, the definition of the derivative. The Reciprocal Rule is defined as
\begin{equation*}
\bigg(\frac{1}{f}\bigg)' (x) = \frac{-f'(x)}{(f(x))^2}.
\end{equation*}
We will prove that this equality holds.
Proof of Reciprocal Rule Derivative using First Principle of Derivatives
\begin{align*}
\bigg(\frac{1}{f}\bigg)' (x) &= \lim_{h \rightarrow 0} \frac{\frac{1}{f(x + h)} - \frac{1}{f(x)}}{h} \\
&= \lim_{h \rightarrow 0} \frac{\frac{f(x) - f(x + h)}{f(x + h)f(x)}}{h} \\
&= \lim_{h \rightarrow 0} \frac{(- f(x+h) + f(x))}{h} \cdot \lim_{h \rightarrow 0} \frac{1}{f(x + h)f(x)} \\
&= - \lim_{h \rightarrow 0} \frac{(f(x+h) - f(x))}{h} \cdot \lim_{h \rightarrow 0} \frac{1}{f(x + h)f(x)} \\
&= f'(x) \cdot \lim_{h \rightarrow 0} \frac{1}{f(x + h)f(x)} \\
&= f'(x) \cdot \frac{1}{f(x)f(x)} \\
&= \frac{-f'(x)}{(f(x))^2}.
\end{align*}
This shows that the Reciprocal Rule indeed holds, which completes the proof.